The Lingo! A car designed for linguists... by linguists—Psammeticus Motors SpecGram Vol CLII, No 1 Contents Masyu Ortograpiu—Trey Jones

Cartoon Theories of Linguistics
Part I—Non-Configurational Languages

Phineas Q. Phlogiston, Ph.D.
Unintentional University of Lghtnbrgstn

A. Mathematician Friend1
“The English have no respect for their language, and will not teach their children to speak it.”
—George Bernard Shaw
once told me that, in mathematics, it is sometimes said that if you cannot explain the basic outline of a mathematical idea to a bright and interested 10-year-old, then you don’t really understand it yourself. That got me thinking, and I’ve come to a couple of conclusions:

It is generally accepted that math is hard (Davis & Hersh, Friend, Lakoff & Núñez, Lucas, Mac Lane, Teen Talk)—and probably harder than linguistics (Friend, Lakoff & Núñez). So, we should be able to reduce the essence of important linguistic concepts to something we can explain to that bright, interested 10-year-old. In fact, I contend that we can boil the essence right down to something we can explain in a cartoon.3

As a first attempt at such a Cartoon Theory of Linguistics, I offer the following treatise on configurational and non-configurational languages:

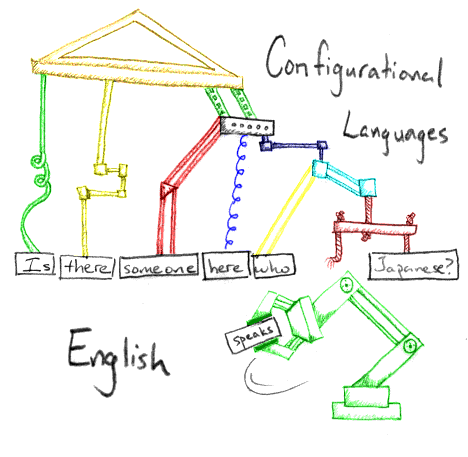
Next time, we’ll look at ergativity.

References
“There is no such thing as the Queen’s English. The property has gone into the hands of a joint stock company and we own the bulk of the shares!”
—Mark Twain
  1. Davis, Philip J., and Hersh, Reuben. (1981). The Mathematical Experience.
  2. Don & III. (1993). "Spaz Attack in the Corner." Speculative Grammarian, Vol. 147, No. 3.
  3. Filipepi, Alessandro. (1988). "Pictures of Lily." Psammeticus Quarterly, Vol. 16, No. 1.
  4. Friend, A. Mathematician. (2006). Personal communication.
  5. Hale, Kenneth L. (1980). "Remarks on Japanese phrase structure: Comments on the Papers on Japanese Syntax." In Y. Otsu & A. Farmer (Eds.), MIT Working Papers in Linguistics (Vol. 2).
  6. Hale, Kenneth L. (1982). "Preliminary remarks on configurationality." In J. Pustejovsky & P. Sells (Eds.), Proceedings of NELS 12 (pp. 86-96).
  7. Hale, Kenneth L. (1989). "On nonconfigurational structures." In L. Marácz & P. Muysken (Eds.), Configurationality: The Typology of Asymmetries (pp. 293-300).
  8. Lakoff, George, and Johnson, Mark. (1999). Philosophy in the Flesh.
  9. Lakoff, George, and Núñez, Rafael. (2000). Where Mathematics Comes From.
  10. Lucas, John Randolph. (2000). The Conceptual Roots of Mathematics.
  11. Mac Lane, Saunders. (1986). Mathematics: Form and Function.
  12. Marácz, L., & Muysken, P. (Eds.). (1989). Configurationality: The Typology of Asymmetries.
  13. Teen Talk, Barbie. (1994). Personal communication.
Notes

1 Not his real name.
2 That you should be able to explain it to a 10-year-old, not that I don’t understand any of them. Damn ambiguity.
3 As luck would have it, this has already been successfully attempted before. See Don & III, and Filipepi.

The Lingo! A car designed for linguists... by linguists—Psammeticus Motors
Masyu Ortograpiu—Trey Jones
SpecGram Vol CLII, No 1 Contents